More about conceptual understanding

One whole or two?

The obvious is sometimes not so obvious. For example, here is a typical fractions addition task.

3/4 + 5/7 = ?

If you are student who "knows what you are doing" then your work may look something like this:

 Since 3/4 = 21/28 and 5/7 = 20/28 then 21/28 + 20/28 = 41/28 = 1 & 13/28

Could the answer ever be: 3/4 + 5/7 = 8/11? "Of course, not," was my gut reaction. But then I read Keith Devlin's* description of how a student can justify this "obviously" wrong answer.

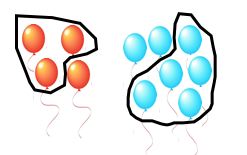
"If I have 4 red balloons of which 1 is burst, than three quarters of my red balloons are good. If I have seven blue balloons and 2 of them are burst, than 5/7 of my blue balloons are good. So what fraction of my balloons are good? Well, altogether I have 4 + 7 = 11 balloons, and of them three are burst, leaving 8 good ones, so the fraction of my balloons that are good is 8/11."

Devlin continues:

"This is perfectly correct reasoning, showing that the student understands the concept of fractions. The students problem is that he or she is unaware that, for very good reasons, the mathematical community long-ago decided that adding fractions mean something different from adding proportions even though fractions may be and are used to quantify proportions." 

Note how the "whole" changes from the first context to the next. So as the saying goes: Keep your eye not on the doughnut, but on the whole when doing fractions.

*Devlin, Keith. "Mathematics Education for a New Era Video Games as a Medium for Learning" p. 68.

The purpose of school (and what it should be)

is to keep you coming every day whether you are learning anything or not. We know that school is designed in such a way that allows adults to do adult-like things and feel comfortable about the safety of their children confined in "day care" centers where sometimes useful learning does break out. But that's not the main goal of schools  rather it is to teach you subject matter using an instructionist approach. Oh yes, you do learn a lot of stuff along the math, science, social studies, history etc. paths that you take. Stuff that for the most part students are not particularly interested in, but they go through the motions to please their teachers and get (or at least try to get) good grades to please their parents. Those were my paths in school and the rites that I accumlated served me well. As least that's what I thought. My teachers and parents were pleased. I did my job well for them. But as an adult I wondered what I would have grown up to become if schools were encouraging me to follow my paths, not theirs. For example, as a kid I was a baseball fanatic. Growing up in Pittsburgh I watched the Pirates transform from a hapless collection of misfit players that were in or near the cellar of their division in the early and mid fifties to a world champion in 1960 to the chagrin of the New York Yankees no less. My passion for baseball served me well in math class. Knowing what was on the back of every baseball card and impressing my 4th grade teacher with how fluent I was in determining the pitcher's earned run average which unbeknowst to me at the time required propotional reasoning which I really didn't get till I became a math teacher many years later. But I was a good parrot. Since I could remember everything related to baseball statistics, I parlayed that skill into success in math class. I mastered all the rudimentary math without really understanding it. But that was good enough to keep me in As throughout my pre-college years. I knew what to do to get the right answers. But what did I really know about math? Not a lot.

This all changed when I became a math teacher and started to learn math from the "outside in*" and fall in love with it for the first time. (Just being good at something doesn't mean you love it.**) -to be continued.

*Baseball is good example of learning math from the outside in. My love of baseball inspired me to learn math since it was an intrinsic part of the fabric of the game.

**Terry Bradshaw Pittsburgh Steeler hall of fame quarterback with 4 super bowl rings was usually depressed before games and had anxiety attacks afterward. In the speeches he gives today Bradshaw invites audiences to think in new ways about sacrifice, pain, competition and adversity, while giving examples of how to focus the power of dreaming, thinking and strategizing towards goals and success. 

Ihor is currently working on a book entitled "The Wannado Curriculum: Scenes from a Dynamic Math Classroom" which is scheduled to be out in 2013. This blog entry is from the initial draft of the book. Comments are most welcome.

A fresh look at the new math days of the late 50s and early 60s

Why does today's new math look like the old math repainted? Back in 1969 Morris Kline had a problem with what was called the new math at that time. Here's what he wrote in chapter 1 of his seminal work "Why Johnny can't Add."

"Evidently the class is not doing too well and so the teacher tries a simpler question. "Is 7 a number?" The students, taken aback by the simplicity of the question, hardly deem it necessary to answer; but the sheer habit of obedience causes them to reply affirmatively. The teacher is aghast. "If I asked you who you are, what would you say?"
The students are now wary of replying, but one more courageous youngster does do so: "I am Robert Sinith."
The teacher looks incredulous and says chidingly, "You mean that you are the name Robert Smith? Of course not. You are a person and your name is Robert Smith. Now let us get back to my original question: Is 7 a number? 0f course notl It is the name of a number. 5 + 2, 6 + 1, and 8 - 1 are names for the same number. The symbol 7 is a numeral for the number."

Technically the teacher is right. 7 IS a numeral that represents the idea of "7ness" meaning 7 of something: an abstraction. Though accurate it was way beyond the call of a beginning student's duty to need to contemplate such subtlety. Though a bit of a stretch sometimes this kind of distinction gets play time when discussing traditional pure math and the necessity for rigor like in the numeral/number distinction. Rigor has its time and place. But not when you want students to have creative adventures in discovering powerful ideas about math or even having students invent their own math. There is a big distinction between what a professional mathematician would consider "inventing new math" and what an average student might do to behave like a mathematician. I discovered that distinction when I personally discovered Pick's Law. Of course, I didn't invent it; it was already a part of mathematical lore thanks to George Pick and Hugo Steinhaus. What I did was recreate it from scratch and that adventure was very meaningful for me. I was now a "mathematician" actually doing math (albeit not inventing it) and certainly not just practicing ideas that the teacher insisted I know whether it was currently relevant or not. I was on a mathematical journey where I made up the path as I went along. The endpoint was defined, but I had to determine the details of the journey following clues along the way. This to me is the new "new math" not an extension with a fresh coat of paint but something all together different. Something that is lacking in most classroom enviroments. I will be sharing some adventures (examples of what I mean) that I've been thinking about in future blog entries.

Levels of Looking at Learning: Cena's fragile knowledge about place value

Keith Devlin writes in his recent blog "How to design video games that support good math learning: Level 4":

"A major problem with video games, or more generally any mechanized educational delivery system, is that the system has no way of knowing what the player, or student, is learning. That a player who moves up a level in a video game has learned something is clear. Video games are all about learning. But all you can reliably conclude from a player’s leveling up is that she or he has leveled up. It could have been happenstance."

The student may not have learned anything significant. Appearances can be deceiving as Keith noted after he watched this video.

Keith continues:

"If you are like me the first time I saw this video, when you heard Cena’s answer in the class you concluded that she understood place value representation. She certainly gave the right answer. Moreover, to those of us who do understand place-value, her verbally articulated reasoning indicated she had conceptual understanding. But she had nothing of the kind, as the subsequent interview made clear." 

Let's review the video above. Here's what happens.

The teacher drew 49 stars and circled 4 groups of 10. When she got to the last 9 stars, she asked the class whether they should be grouped? 

"No," says Cena. "You got uhh one, two, three, four tens. You like put a four right there. And you have 9 stars left over so you put 9 right there." 

To which the teacher replies, "So does everyone understand?"

At that moment Marilyn Burns comes on and says, "Children's understanding is often fragile but what they know in one setting doesn't always transfer to another."

New scenario. Marilyn is now sitting next to Cena with a bunch of tiles on the table. Marilyn continues, "Put the tiles in groups of 10 and count out loud so I hear what you are doing." Cena counts out ten as she places them in a pile. "Can you make another pile?" Marilyn continues. "So how many groups of 10 do you have? "2," Cena responds. how many more do you have? "4". Do you know how many tiles you have all together? Cena responds with "Uh-uh (no)."

How is that possible? This is the same girl that so brilliantly knew to write that there were 49 stars by counting 4 groups of 10 with 9 left over. Keith didn't offer a possible explanation and neither did Marilyn. That, of course, was not their purpose; all they wanted was to make the reader/viewer aware that what you see is not necessarily what you get.  So that begs the question for me. What would you do: (1) as the teacher in the classroom to confirm Cena's understanding and (2) as a tutor ala Marilyn Burns?

Cool Graph about the Growth of Charter Schools

click on image for animation

Whether you like them or not Charter Schools are here to stay. If we want our kids to have 21st century skills then they need schools that will help our students achieve them. Ronald Wolk in his book "Wasting Minds: Why our education system is failing us and what we can do about it" writes that what we need is in addition to reforming our existing schools is a parallel strategy of alternative schools focusing on creativity and innovation.  He says: 

"Standards-based accountability is here to stay – at least for a long time. So the only other rational response I can think of is to adopt a parallel strategy and pursue it simultaneously – what my friend Ted Kolderie (2010) calls 'a split screen' approach to improving education. [Link] Why should we bet everything on a single strategy, especially if it isn't working? Why not have at least one alternative strategy? Why not have parallel strategies that seek to achieve the same objectives? Why can't we walk and chew gum at the same time?" (Wolk, 2010)

Wolk holds promise for innovation in the marriage of technology with chartering. He says "Chartering and the Web, though still fledgling efforts, have laid the foundations for a second strategy of creating new educational opportunities that put students and learning first." (Wolk, 2010) Sounds good to me. Now how would that work? The Wanna do curriculum movement (see my book proposal) will be one positive step in that direction.

Kolderie, T (2010, April). Innovation-based systemic reform: Getting beyond traditional school. Education Evolving. Retrieved from http://educationevolving.org/pdf/Innovation-Based-Systemic-Reform.pdf

A New Journey Begins

Welcome to my other blog. Starting in September this will become my main blog and CLIME Connections will then be my other blog. Why do I want to put it on the backburner? Well, I've been involved with CLIME since 1986 and it's time for me to move on. Not immediately. I'll be stepping down as president and proprietor of the CLIME organization at the end of the 2012-2013 academic year.
One of the goals I wanted to accomplish after I retired from Stevens/CIESE in 2007 was to help put Web 2.0 on the math teacher's radar screen as they plan for their student's learning experiences. Last April at the NCTM meeting in Philadelphia I had a revelation that the math community had turned a corner and Web 2.0 had made its imprint. For the first time, at a annual NCTM conference attendees had free Wifi access (when it was working properly) almost everywhere in the convention center. So for the first time it made sense for a conference attendee to carry a smart device (iPhone, Android, laptop etc.) with them to the conference. NCTM made a free app available so you didn't even need to carry around the annoying program book that's never convenient when you really need it or worse if you lose the book which I'm prone to do. For the first time NCTM has sent a message that ubiquitious connectivity is the future not only for conference going but also in schools where taking advantage of this new power with students and using it effectively is the ultimate 21st century challenge.  How my vision for this new Dynamic Math Classroom as I call it plays out is what I'll be writing about in this blog.

Next: Math 2.0 - Scenes from a Dynamic Classroom - the book.

Average Traveler Activity Redux

At the NCTM Conference last April I did what I call the Average Traveler activity with the 36 math educators who attended my session "Math 2.0: Scenes from a Dynamic Classroom."
Based on the distances that each attendee traveled to get to this conference, I asked who in this room would represent the average distance traveled? Guesses ranged from 200 to 800 miles. Since we didn’t have access to computers to do this in real time, I used the distance from each attendee’s school (or administrative office) to the Convention Center in Philadelphia, PA (where this session took place) to figure this out. I added placemarks at each school's location using Google Maps and used the distances provided by the software. Which school’s placemark do you think is closest to the average distance that the participants traveled to this conference? The yellow marker is the site of the conference.

I used Geometer’s Sketchpad to draw a circle with the location of the session at the center and the radius of the circle gives an estimate of the distance to the various schools represented. By changing the radius of the circle I could approximate what the average was. The radius of the circle in the image is 809 miles which is the approximate value for the average. Note there are no schools that are candidates for being closer to the average distance than Mr. Fogg. Take a look at Google Maps and identify your location. The sites are open for you to make corrections and updates.

Here is a Google spreadsheet of the relevant data.

Please post your comments or questions about this activity below.

In Search for the Last Digit of Pi

Fig. 1

Ever wonder what the last digit of Pi is? Well, if you are one of those in the know, you know that there is no such thing. Pi is irrational. Right? And you know that the decimal expansion of Pi (3.14159...) goes on forever without any recurring patterns. 

Fig. 2

But what about this headline back in 2004? 

Did this really happen? Read the rest of the article by clicking on it. What do you think?

"The Last digit of Pi" is the title of Dan Cohen's very interesting blog about how some people passionately pursue the last digit despite knowing that they will fail. It's also a nice story about the evolution of knowledge about Pi. He also has done an entertaining TED talk on the topic. 

Sunday Morning (CBS) highlights Pi Day

This a recent segment about Pi day.

For more Pi Day resources see my Pi Day page.

My favorite Pi Day activity is the Buffon Needle Experiment. See my lesson. See also this link. See also Matthew Blake's cool simulation of the needle experiment.

Here we go again

Latest issue of Summing Up

Latest "NCTM Summing Up" posts the inevitable. More study needed. What a surprise. As if we haven't done enough studies already! It's business as usual. The major players in the math ed community want to have fun telling us what we should be doing with our students using scare tactics to get the nervous public to go along with their latest hype. We're still a "Nation at Risk" and will continue to be as long as the "hafta-do" curriculum rules in most schools. Kids need to be heard more and college professors less because the current paradigm is not making any substantial improvements in teaching school math.